How is a quantum bit like a ballerina?

Having a single point in contact with the ground lets a ballerina spin. In the same way, a quantum state is dynamic because it can turn about a point thanks to quantum uncertainty, say CQT and Oxford researchers. Photo: Michael Garner, courtesy English National Ballet.

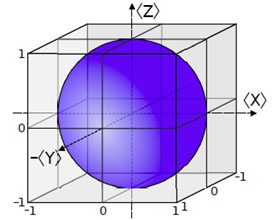
The possible states of a simple quantum particle are described by the 'Bloch sphere'. In theories without an uncertainty principle, states could fill the whole cube.

When a ballerina pirouettes, they balance on the very tip of the toes of one foot. This allows them to spin freely. Singapore and Oxford researchers suggest a quantum state does something similar, drawing the analogy to explain a connection between the ability of a quantum state to evolve and the quantum uncertainty principle. The researchers present this connection in an 8 August paper in Nature Comunications.

The uncertainty principle is one of the most famous tenets of quantum theory. The principle holds that you can't know everything about a system at once. A particle, for example, has pairs of properties that cannot be known simultaneously with complete precision.

The idea is nearly a century old, but it still has researchers puzzling. It's uncomfortable to think that the universe is fuzzy at its most fundamental level. Why might this be?

Oscar Dahlsten, Andrew Garner and Vlatko Vedral came up with an intriguing answer by looking at the impact of uncertainty on a particle that is restricted to being in one of two places. Vlatko is a CQT Principal Investigator and Oscar a former CQT Research Fellow. They and Andrew are also at Oxford University, UK.

The two-position particle, like a quantum bit, is pictured by physicists as a 'Bloch sphere' (see figure). The North and South poles represent the particle being at site A or B respectively. In classical physics, the points at the poles are the only states that exist: the particle is always either at site A or site B. But a quantum particle can be at A with some probability and at B with some probability. This quantum state has 'shape' because a full description needs not only the position information but also 'phases' associated with each site – these are subtly hidden numbers that aren't there in classical mechanics and which give rise to phenomena such as quantum interference.

Uncertainty gives the quantum particle its spherical shape because it governs position and phase: the more well-defined the position, the less well-defined the phase, and vice-versa.

But it's possible to imagine theories that have phases and certainty. In the most extreme case, the Bloch diagram could be cube-shaped, with points in the corners corresponding to particles with definite everything. Researchers call this hypothetical scenario 'box-world'.

Oscar, Andrew and Vlatko find that the extra certainty of box-world has dramatic consequences. In fact, they show that such states would end up frozen, their phases unable to change at all! "We show that if a theory has such extra numbers it needs to have something like the uncertainty principle in order for these to be alterable, ie in order for non-classical dynamics to exist," says Oscar.

To reach this insight, the researchers imagined doing something at site A to affect particles at site A. They suppose that this cannot affect a particle if it is definitely known to be at site B. "Contrary to some popular science depictions, quantum theory does not allow action at a distance. The universe would be almost inconceivably odd and complicated if action at a distance were possible," says Oscar. Effectively, this means that operations at site A fix all points in the opposite plane, corresponding to particles existing at site B, and vice versa.

"It is like the points are stuck by total friction. This means the cube has a big disadvantage, it gets stuck and no states can change. But imagine metamorphosing the cube into a sphere, or indeed something else with only one point on the lower plane, like how the ballerina goes up on one toe. Then the shape, with all the quantum states in it, can move," explains Oscar. Operations at one site can alter phases by 'spinning' the Bloch sphere about the single point fixed in the opposite plane. To put it another way: the uncertainty principle is liberating, not limiting.